Lunda Designs

      THE
DISCOVERY
       OF
   LUNDA
DESIGNS

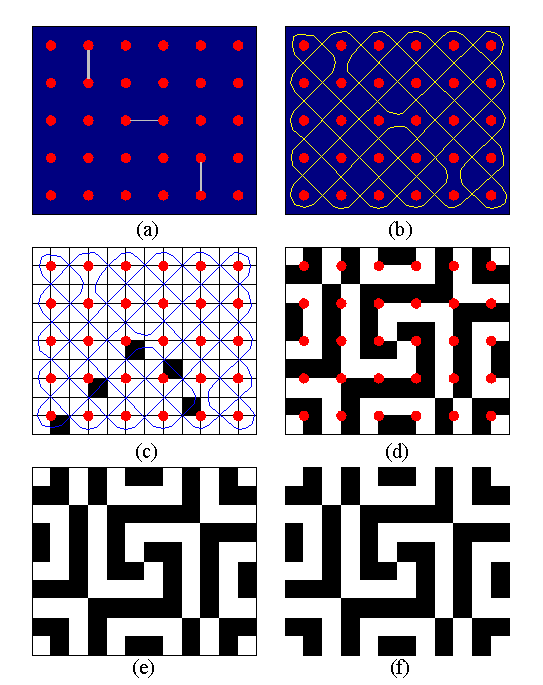
Let us now consider a rectangle-filling mirror curve. It passes precisely once through each of the unit squares of the rectangular grid. This enables us to enumerate the unit squares through which the curve successively passes, 1, 2, 3, 4,...,4mn. Enumerating them modulo 2, i.e. 1, 0, 1, 0, ..., 0, a (1,0)-matrix is obtained, or, equivalently, by colouring the successive unit squares alternately black (= 1) and white (= 0), a black-and-white design is produced. Figure 7 presents an example of the generation of such a black-and- white design.

Figure 7: Example of a black -and-white colouring. (a) Mirror design; (b) Corresponding mirror curve; (c) Starting the colouring; (d) Final black-and-white pattern (with the grid points marked); (e) Final black-and-white pattern (with the grid points unmarked); (f) Final black-and-white pattern (with the border rectangle unmarked).

As this type of black-and-white design was discovered in the context of analysing sand drawings from the Tchokwe, who predominantly inhabit the north-eastern part of Angola, a region called Lunda, I have given them the name of Lunda-designs.

NEXT